My notes on research methods and studies in teaching and learning mathematics
Teachers don’t merely deliver the curriculum. They develop, define it, and reinterpret it too. It is what teachers think, what teachers believe and what teachers do at the level of the classroom that ultimately shapes the kind of learning that young people get. (Hargreaves 1994, p. ix)
Hargreaves, A. (1994). Changing teachers, changing times: teachers’ work and culture in the postmodern age. London: Cassell.
It was Shulman in 1986 who first thought and recognized that the nature of knowledge for teaching a subject matter is different from knowledge of the subject matter itself (content knowledge or CK) and from general knowledge of pedagogy. He introduced the phrase pedagogical content knowledge (PCK) to describe the blending of content and pedagogy. He describes PCK as follows:
Within the category of pedagogical content knowledge, I include, for the most regularly taught topics in one subject area, the most useful forms of representation of those ideas, the most powerful analogies, illustrations, examples, explanations, and demonstrations – in a word, the ways of representing and formulating the subject that makes it comprehensible to others. (1986, p.9)
Shulman’s description of knowledge needed by a teacher have been developed further. In mathematics, Ball and Bass (2003) introduced the notion of mathematical knowledge for teaching (MKT) which is similar to PCK and they include the idea of ‘unpacking’. Ball and Bass argued that in advance mathematical work, knowledge is ‘compressed’ and that the teachers work is to ‘decompress’ or ‘unpack’ this knowledge for their students.
Bernardz and Proulx (2009) also characterized mathematics for teaching knowledge as knowledge-in-action or knowing-to-act (as opposed to factual knowledge) and that it is situated since it develops in a specific context linked to a practice in mathematics teaching and is not independent of students’ learning, of the classroom, etc. They describe the work of a teachers as:
The teacher must constantly reflect on possibilities, offer and invent new avenues and representations in reaction to students’ action, think of additional explanations to clarify or re situate the tasks offered, choose to emphasize some aspects and not others, know that this or that explanation or representation is related to what the student offered as a solution and may eventually benefit this students’s understanding, etc.
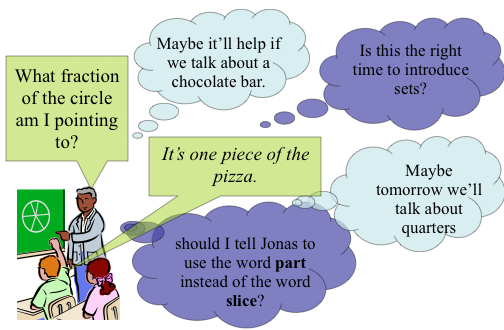
Here’s a picture that will illustrate this: (I’m still looking for the powerpoint in slideshare where I got this picture)
Reference
Knowing and Using Mathematics in Teaching: Conceptual and Epistemological ClarificationsAuthor(s): Nadine Bednarz and Jérôme ProulxReviewed work(s):Source: For the Learning of Mathematics, Vol. 29, No. 3.
